Fitting a Quadratic Function to Data

Example: Problem #75 on page 273 from the text titled “College Algebra: Graphs & Models”, Third Edition, by Bittinger, Beecher, Ellenbogen, and Penna. Fit a quadratic Function to the Data shown in the table below. Let x represent the number of years since 1992.

Year	Mortgage Debt (In Billions)
1992	$4254
1993	4209
1994	4381
1995	4577
1996	4865
1997	5203
1998	5723
1999	6360
2000	6887
2001	7596

Before you begin make sure that the Plot1 is ON. Make sure that you clear out any functions that may be stored in the function editor. Your screens should look like the ones shown in Fig.1 and Fig.2. You might want to review the worksheet, Preparing the Calculator for Regression.

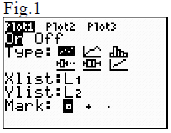
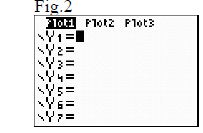
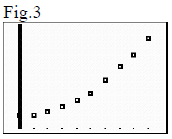
To begin, press , choose for EDIT. Enter all the x-values in the L1 column and all the y-values in the L2 column using the same steps as you did when fitting a linear function to data. Once all the data is entered, press Your screen should look like the one shown in Fig.3.

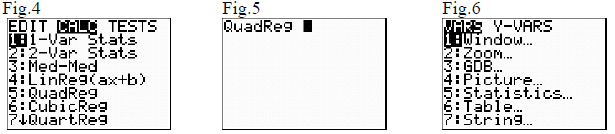
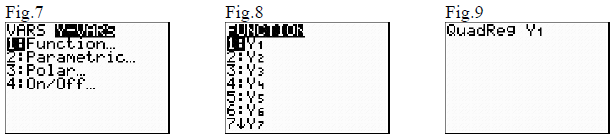
Next, press so that your screen looks like the one shown in Fig.4. Select to get the screen shown in Fig.5. Press resulting in the screen shown in Fig.6. Press . Your screen will look like the one in Fig.7. Press to see the screen in Fig.8. Press , then again resulting in the screens shown in Fig.9 and Fig.10 respectively.

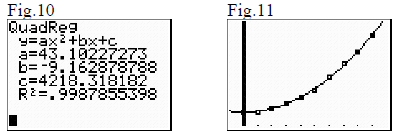
In Fig.10 you will see that a = 43.1, b = -9.2, and c = 4218.3, rounded to the nearest tenth. Press to graph the quadratic function that you have calculated. The result is shown in Fig. 11.

The quadratic function, f(x) = ax² + bx + c, that best fits the data is f(x) = 43.1x² - 9.2x + 4218.3.

Note: To find the cubic or quartic function that best fits a set of data you will follow the same procedure as for finding the quadratic function. When you get to the screen shown in Fig.4 you would select 6: CubicReg for the cubic function or choose 7: QuartReg for the quartic function.